apt4Maths: Set of 14 PowerPoint Presentations (Relating to Geometry and Measures) on Shapes, Angles and Constructions for GCSE (and Key Stage 3) Mathematics

Product Information

This set of 14 PowerPoint Presentations (238 slides, excluding Title Pages) covers the following topics: 

• 01 Shapes – Terminology and Properties (18 slides): Defines key terms relating to shapes and angles and explains the types and properties of triangles and quadrilaterals.

• 02 Angles – An Introduction (25 slides): Explains key words and facts linked with turning and position, the different type and sizes of angles, how to estimate the size of angles, and how to measure angles accurately.

• 03 Angles – Simple Facts (20 slides): Explains the terms complementary, supplementary, and vertically opposite (in the context of angles), and explains how to find missing angles within right angles, on straight lines, around a point, inside triangles and inside quadrilaterals.

• 04 Angles – Polygons (20 slides): Reviews the names of common polygons, explains how to calculate exterior and interior angles of regular polygons as well as missing angles of irregular polygons, and explains what is meant by the term ‘tessellation’.

• 05 Angles – Parallels (23 slides): Explains the types of angles formed when a transversal line crosses parallel lines and explains how this knowledge can be used to calculate missing angles.

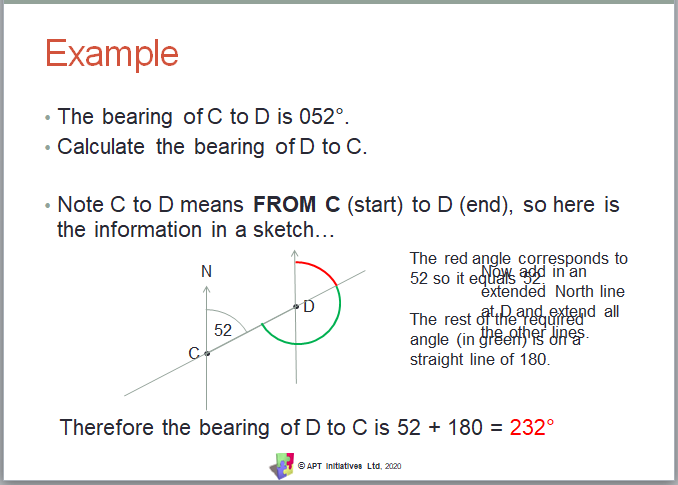
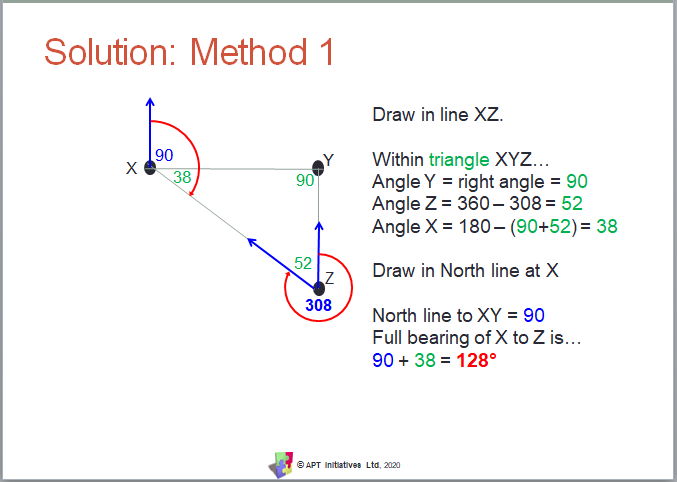
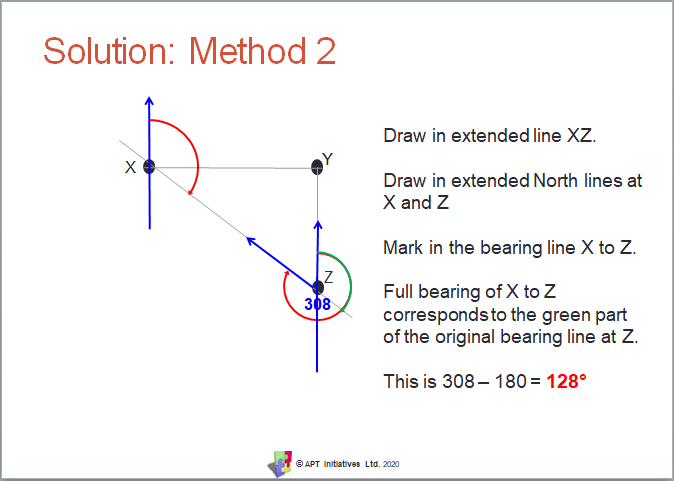
• 06 Angles – Bearings (9 slides): Explains how to write, measure, and calculate bearings, and reviews and uses prior knowledge of angles at parallel lines.

• 07 Circle Theorems (21 slides): Reviews terms linked to circles before explaining the eight circle theorems and how these can be applied to find the size of angles.

• 08 Basic Trigonometry (24 slides): Explains: how to label the sides of a right-angled triangle for trigonometry; the names of the 3 main trigonometric ratios (sine, cosine and tangent); how to find missing sides and missing angles in right-angled triangles; the trigonometric ratios for 30°, 45°, 60° and 90°.

• 09 Sine Rule (14 slides): Explains the Sine Rule and how to use it to find missing sides and angles, and practises manipulating formulas, particularly ones given in fraction form.

• 10 Scale Drawings (11 slides): Explains how to work out distances required on a scale diagram, model or map, and how to work out the real distances represented by a scale diagram, model or on a map.

• 11 Constructions (13 slides): Explains how to construct SAS, ASA, and SSS triangles, and how to construct angles (of 90°, 60°, 45° and 30°) without using a protractor.

• 12 Bisectors (11 slides): Explains how to bisect lines at right angles and how to bisect angles.

• 13 Locus (14 slides): Explains what is meant by a locus, reviews perpendicular bisectors of lines and angles of bisectors, and explains how to find the locus of a given situation (using prior knowledge).

• 14 Views (15 slides): Explains 2D representations of 3D shapes, and explains different 2D views (front, side and back elevations, and plans) of 3D situations.

Note:

• Work on Pythagoras’ Theorem, the Cosine Rule, and the trigonometric formula for the area of a triangle, is included in APT’s set of PowerPoints on ‘Measures, Perimeter, Area & Volume’.